3.10 Special Rules and Scales
The above sections describe most of the primary scales that can be found on typical slide rules. A basic slide rule might have a limited number of scales, such as those found on the Mannheim layout. Some of the more advanced slide rules might have 10-20 scales on them, with folded scales and log-log scales and perhaps hyperbolic trig scales. However, similar to how a computer program or application might be used today, many other specialty slide rules also were developed for particular types of repetitive calculations found in various professions.
3.10.1 Specialty Rules
An example of a specialty rule would be an “Electro” slide rule, which is a type that was made by various manufacturers for the specific use in the electrical trades. It has Gauge Marks on certain scales corresponding to the resistivity of copper, assuming diameters of wire in inches or millimeters, for instance, and lengths in feet or meters. Knowing the standard voltage used in the system, voltage drops, currents, and power levels can be computed quickly.
Other examples include “chemical” slide rules with built-in marks indicating commonly used densities and other properties of various compounds for use in chemistry; “radio” and “electronics” rules used in the radio and electrical engineering industries with special scales for relationships between frequencies, capacitance, decibels, and other common variables; Stadia Rules used in surveying, which provided direct calculations of horizontal offset and vertical rise from transit readings; “artillery” rules used in the military; “proportions” rules used in typography and engraving applications, and so forth.
In many cases the Specialty Slide Rules can be quite rare and are often highly sought after by collectors. (Personally, I’d love to have a Black Body radiation slide rule!)


3.10.2 Long Scales
To complete our tour of the subject, there are slide rules with basic scale arrangements but with very long scales for reading values to more significant figures. Some of these are themselves quite long – 20 inch Mannheim-type rules were common in the early part of the 20th century. But circular slide rules also can give higher accuracy with scales that can be up to a factor of \(\pi\) longer than their overall dimension. Spiral scales (such as on the Boykin RotaRule and even helical scales (such as the Otis King’s Calculator and the Fuller Calculator) can give total scale lengths of up to several feet, providing multiplications and division with impressive accuracy. Examples can be found in the section Non-Linear and Long-Scale Rules. See also the vignette, The Long and Short of It.
3.10.3 Segmented Scales
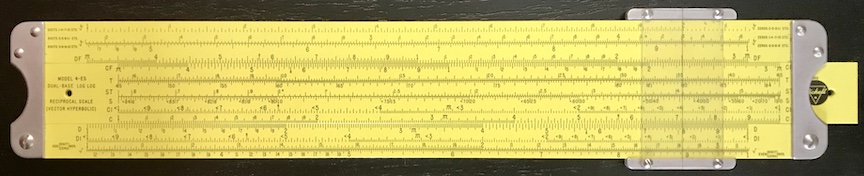
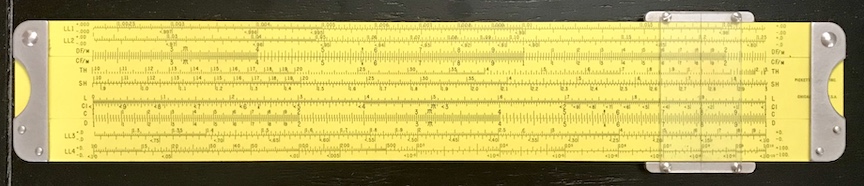
Some of the more modern rules have certain scales that are broken up into segments, so that a 20-inch scale can fit onto a 10-inch rule, for instance. One of my favorite slide rules is the Pickett Model 4. (See figure below.) It has the following scales on it:
Front:
- a 30-inch equivalent cube/cube-root scale
- a 20-inch equivalent square/square-root scale
- 20-inch equivalent T and S/ST scales
- standard 10-inch C/D, CI/DI, CF/DF scales
Back:
- standard 10-inch C/D, CI scales
- CFm/DFm scales – folded at value of \(\ln 10\) for conversion between Base \(e\) and Base 10
- 80-inch equivalent log-log scale
- TH and SH scales for direct reading of hyperbolic trigonometric functions and direct vector calculations
And all in Eye-Saver yellow with black text!